The T600 was designed to show the potential of an ultra-narrow commuter car. It was designed to overcome each potential objection with extreme examples of acceleration, cornering, etc. Hence, we designed it with an unprecedented amount of torque for a street car. It uses the same kind of motors as freight train locomotives, having a staring torque of over 1,500 ft-lbs each. The combined torque of these motors, starting at zero RPM, is 3,000 ft-lbs, the equivalent of 6 Dodge Viper V-10 engines, 8 Ferraris, or several semi truck tractors engines.

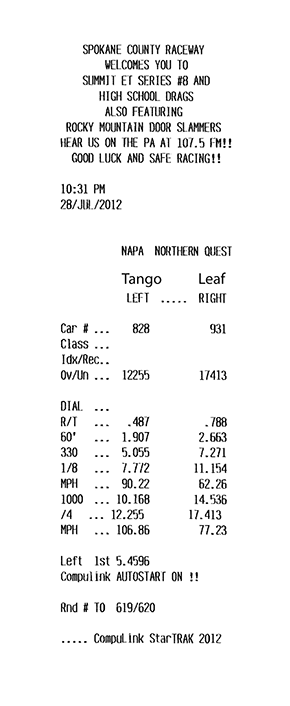
Because of the extreme torque these series-wound motors have, the Tango needs only one gear to go from zero to about 150 mph. That gear, a 3:1 ratio from motors to axles, allows it to accelerate from 0 to 60 mph in 3.2 seconds, finish the standing ¼ mile in 12.25 seconds at 106 mph, and do a wheel stand if given enough traction. Redline on the motors in 8,000 rpm which would equate to  172 mph.

The added benefit to using these high torque motors is that no gear shifting is necessary. It also eliminates a differential and complex gearbox, allowing the entire drive-train to fit between the rear wheels, leaving more space for the battery, hence more range. Contrary to common belief, the large motors do not use more power than small motors, unless the horsepower is used. Unlike an internal combustion engine (ICE), there is no extra friction due to size, so the motors run virtually just as efficiently as smaller ones that are doing the same job, for example driving at 60 mph on the highway. At higher speeds on the freeway, the Tango is actually much more efficient than the Nissan Leaf with its AC drive system. Some of this may be due, however, to the reduced wind resistance because of the Tango's smaller profile.

Although the torque is off the chart, the horsepower is not as remarkable. Horsepower = Torque (in ft-lbs) times RPM divided by 5,252. These series-wound traction motors start out at their highest torque which then diminishes with RPM. The motor controller can put 2,000 amps at over 300 volts to the motors. That's 600 kW = 805 hp. The motors, however, won't accept this current at higher RPM. To remedy this problem of back EMF, which is the increasing resistance developed by the spinning motors, we have a shift from a series connection to the motors, to parallel. This gives a boost, much like a passing gear when going over 60 mph and needing to pass a car quickly.

The torque at the motors is proven in the video below. There you can see the front wheels lifting off of the ground. Simple high-school level physics will allow one to calculate the torque based on these facts. The Tango weighs 3,326 lbs. There is a 43% front and 57% rear weight bias. From that you can calculate that it would take 9,000 ft-lbs on a torque wrench attached to the rear axle in order to lift the front wheels off of the ground. With a 3:1 gear ratio it has to have 3,000 ft-lbs at the motors. When that happened on well-prepared drag strip, that gave the Tango enough traction not to spin the tires, the motor torque was proven.